If you’re interested in Wolfram Physics, you’ve probably heard of John von Neumann.

For a few decades of the early twentieth century, every time there was an important discovery in physics, mathematics or computation, John von Neumann was there.
It’s as if he were the biggest photobomber in Wolfram Physics prehistory.
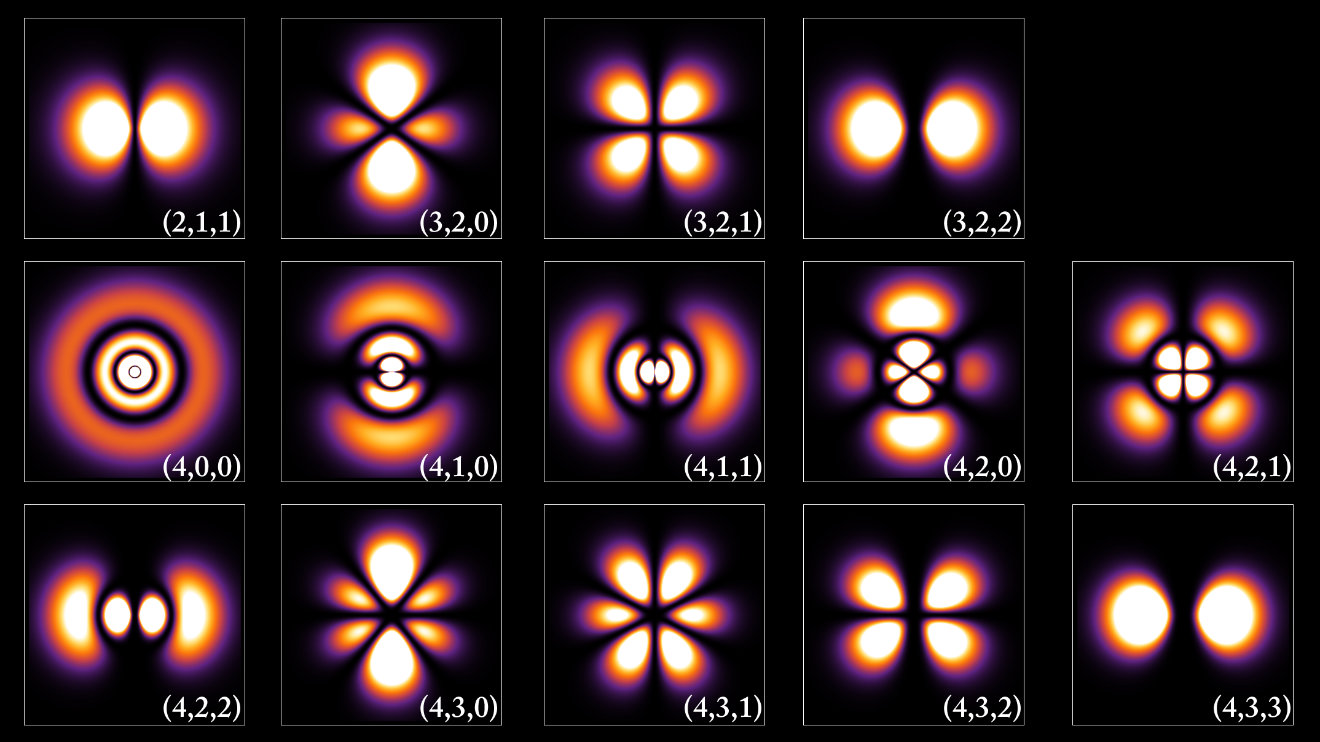
The discovery of quantum mechanics? John von Neumann was there.

Gödel’s theorem? John von Neumann was there.

Turing machines? John von Neumann was there.

The Manhattan Project? John von Neumann was there.
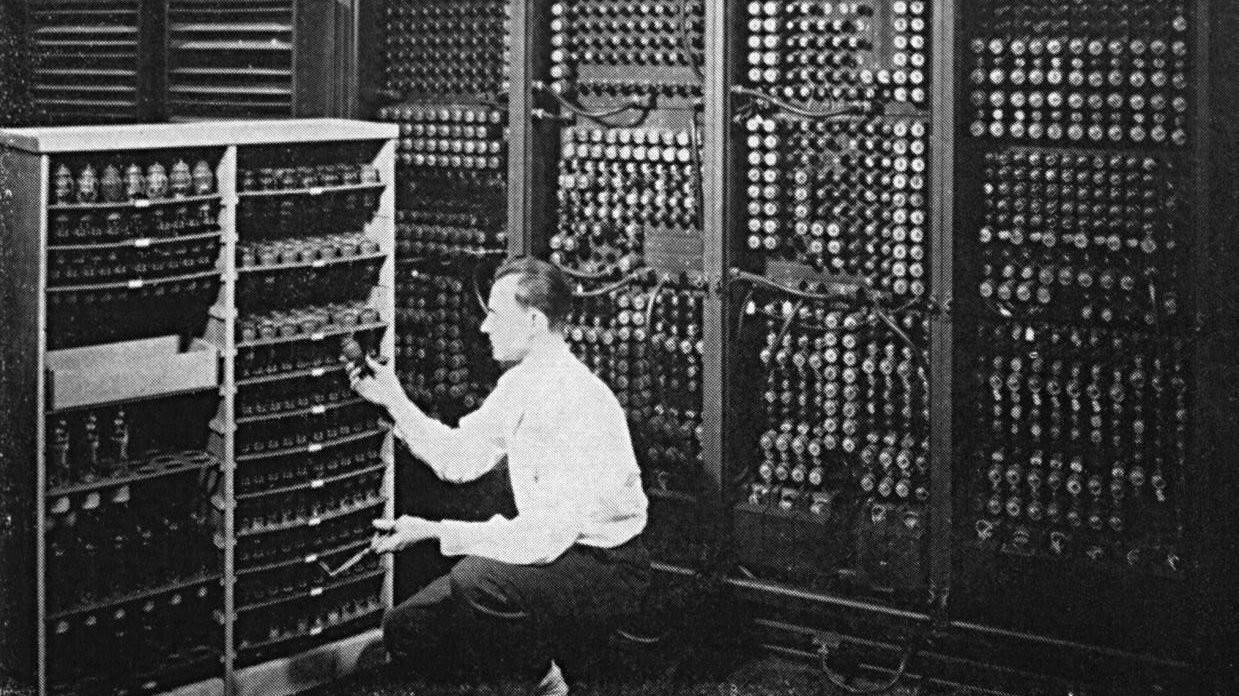
The invention of the electronic computer? John von Neumann was there.
The development of cellular automata? John von Neumann was there.
And yet, if you’re not interested in these prerequisites to Wolfram Physics, you might never have heard of John von Neumann.

Einstein? Of course you’ve heard of him.

Schrödinger? Well, if you haven’t heard of him, you’ll at least of heard of his cat.

Heisenberg? Certainly.

Gödel? Completely.

Turing? Again, if you haven’t heard of him, you’ll at least have heard of his test.
But von Neumann? Maybe not.
Who was John von Neumann?
And how has someone who made such extraordinary contributions to physics, mathematics and computation remained relatively unknown?
For this, another high-budget, big-hair episode of The Last Theory, I flew all the way to Budapest, where John von Neumann was born, to point to a plaque and get some answers.

Quantum mechanics
In the 1920s, physicists were putting together a very strange theory of very small things.

Heisenberg’s version of the theory used matrices: infinite arrays of numbers corresponding to the frequencies of light emitted by atoms.

Schrödinger’s version of the theory used waves: invisible fields of probability corresponding to the chances of a particular particle being in a particular place at a particular time.
Guess who was there to resolve these two different approaches?

John von Neumann.
He showed that mathematically, Heisenberg’s matrices and Schrödinger’s waves were different formulations of the same theory.
He even provided his own formulation, rigorously deriving quantum mechanics from the properties of Hilbert space.
So why is von Neumann not one of the names we think of when we think of quantum mechanics?
Partly, it’s because he didn’t discover any fundamentally new aspects of the universe.
Heisenberg’s uncertainty principle? You can’t know both the position and the momentum of a particle at the same time? That’s new.
Schrödinger’s probability waves? You can’t predict the outcome of a measurement, only the probabilities of possible outcomes? That’s new.
Von Neumann’s rigorous formulation of these same oddities? Not so new.
Partly, however, we don’t remember von Neumann’s contributions to quantum mechanics because what he did was mathematical.
Let’s face it, no one really understands mathematics. Or, at least, I don’t. Maybe you do? John von Neumann certainly did. But the rest of us?
I mean, seriously, Hilbert space?
A particle that can’t decide whether it’s coming or going, now that’s something I can picture.
A cat that’s half dead and half alive, that’s something I can picture.
Hilbert space? That’s a level of abstraction that’s beyond most of us.
We can’t understand John von Neumann’s mathematics, so we don’t remember his name.
Gödel’s theorem

In 1930, Gödel announced that there are certain mathematical propositions that can’t be proved.
This modest announcement shocked mathematicians. They’d been expecting that some time soon they’d put the finishing touches to a complete and consistent system of mathematics. Gödel had proved that this was impossible.
Actually, at first, Gödel’s announcement didn’t shock anyone. No one seemed to realize that he’d proved the grandest project of mathematics a pipedream.
Guess who was there to recognize the importance of Gödel’s theorem?

John von Neumann.
After talking to Gödel about his methods, John von Neumann worked out the full implications of Gödel’s ideas for the completeness and consistency of mathematics.
So why is von Neumann not the name we think of when we think of this most important of mathematical theorems?
Because Gödel got there first.
By the time John von Neumann sent Gödel his work, Gödel had already worked out the full implications of his own ideas.
It seems right that having had the initial ideas, Gödel should get the credit for the math-shattering implications, too.
But it tells you a lot about John von Neumann that he was the only other mathematician to immediately grasp the importance of Gödel’s theorem, and the only one capable of extending it to its revolutionary end as quickly as Gödel himself.
Turing machines

In 1937, Turing described a machine capable of performing computations by reading and writing symbols on a paper tape.

Turing showed that by interpreting these symbols as instructions, this imaginary machine could simulate any other computer. In other words, a universal Turing machine could perform any computation.
Just as Gödel proved that no system of mathematics can be complete and consistent, so Turing used his thought experiment to show that there’s no systematic way to decide whether a mathematical statement is provable.
Turing’s imaginary machine, with its ability to execute instructions held in memory, was designed to prove the undecidability of mathematics. It was not a blueprint for a real computer.
Guess who was there to turn Turing’s machine into a blueprint for a real computer?

John von Neumann.
Impressed with Turing’s ideas, John von Neumann offered him a position as his academic assistant, an offer Turing turned down.
Years later, however, when John von Neumann outlined a structure for the electronic computer, it included the ability to execute instructions held in memory.
Early computers had held numbers in memory, but instructions had generally been fed in as patterns of holes in punchcards.
The ability to execute instructions held in memory, transforming these early computers into machines capable of any computation, was straight out of Turing’s proof of the undecidability of mathematics.
Why is von Neumann not the name we think of when we think of these foundational concepts in computation?
Because Turing got there first.
Still, John von Neumann is given the credit for turning Turing’s ideas into a blueprint for a real computer. The structure he outlined, used for computers ever since, is known as the von Neumann architecture.
The Manhattan Project
In 1944, the scientists at Los Alamos working to build an atomic bomb had a problem.
The production of uranium was going so slowly that there would barely be enough for a single bomb.
There would be more plutonium available, but it was so fissile that the simple gun design favoured by the leaders of the Manhattan Project wouldn’t work. A bomb that shot a small piece of plutonium into a larger piece would melt before it could reach a critical mass.
Guess who was there to hone the alternative implosion design?

John von Neumann.
As so often in his life, John von Neumann took a concept originated by someone else, in this case Neddermeyer’s implosion design, and ran with it.
He proposed a sphere of explosive lenses that would focus shock waves on a core of plutonium, compressing it into a critical mass.
As the Trinity test and the bombing of Nagasaki demonstrated, John von Neumann’s design worked.

So why is von Neumann not one of the names we think of when we think of the atomic bomb?
In light of the Manhattan Project’s secretiveness, no one beyond the leadership was given much credit for its success; indeed, in light of its destructiveness, no one was especially eager to take the credit.
Electronic computers
The invention of the electronic computer involved so many different innovations from so many different people that no one person could possibly be identified as the inventor.

But guess who was there when the earliest electronic computers were built?

John von Neumann.
Not only was he instrumental in the construction of one of the earliest electronic computers, the IAS machine, completed in 1953, but he was also involved in the design of countless others, including the ENIAC, the EDVAC and the IBM 701.
There were some who thought that John von Neumann got too much of the credit for the electronic computer. In particular, a couple of guys named Eckert and Mauchly who, like von Neumann, had worked on both the ENIAC and the EDVAC, wanted to patent their design and, presumably, prevent anyone else from developing computers without paying them money. Happily for humanity – though unhappily for Eckert’s and Mauchly’s bank accounts – their patent application was thwarted when the director of the IAS machine made public John von Neumann’s account of the design they had all worked on.
Why is von Neumann one of the names we think of when we think of the electronic computer?
Because John von Neumann was more interested in disseminating his inventions than in tying them up with money-grubbing patents.
Cellular automata
Many of the discoveries I’ve mentioned so far – quantum mechanics, Gödel’s theorem, Turing machines, electronic computers – are directly relevant to Wolfram Physics.
But none of these discoveries is so proximate a prerequisite to Wolfram Physics as cellular automata.

When Stephen Wolfram wrote A New Kind of Science in 2002, he focused exclusively on cellular automata. Only later, with Jonathan Gorard and Max Piskunov, did he develop his ideas to encompass hypergraphs.
Cellular automata are grids of cells to which simple rules can be applied to give rise to complex behaviour, including self-replicating sytems that look a little like living organisms.
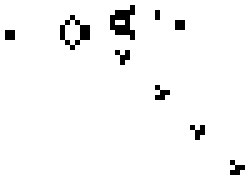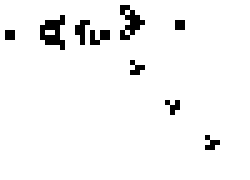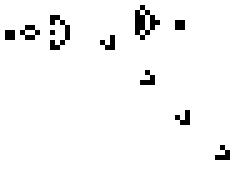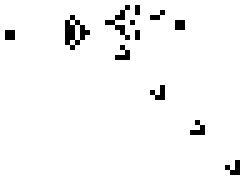
They can be simulated with computers, but the concept of cellular automata was developed in the early 1940s, before the earliest electronic computers were built.
Guess who was there when cellular automata were discovered?

John von Neumann.
When he was working on the Manhattan Project at Los Alamos, John von Neumann’s mind was far too lively to be limited to calculating the shapes of explosives. He indulged in late-night discussions of diverse intellectual topics irrelevant to the making of the atomic bomb.
Why is von Neumann one of the names we think of when we think of cellular automata?
Because, along with with his compatriot and Los Alamos co-worker Ulam, he founded the field.
The art of being there
I’ve touched on only a handful of John von Neumann’s contributions to physics, mathematics and computation. I haven’t even mentioned his extraordinary work in set theory, game theory or artificial intelligence.
John von Neumann was undoubtedly one of the most brilliant minds of the twentieth century.

Yet here in Budapest, the city of his birth, where there are countless monuments to Hungarian leaders, composers, writers, actors and even footballers, the only memorial of John von Neumann is this modest plaque:

A Jesuit priest named Strickland once said: “A man may do an immense deal of good, if he does not care who gets the credit for it.”
(Ironically, variations of this quote are routinely credited to various others who lived later than Father Strickland.)
John von Neumann did an immense deal of good in his lifetime. Sometimes he got the credit for it. Sometimes he didn’t.
It’s true that he did not, by himself, make any of the truly foundational advances in physics, mathematics or computation, for which the likes of Einstein, Schrödinger, Heisenberg, Gödel and Turing are rightly remembered.
But he always seemed to be there when it happened.
John von Neumann had a rare ability to recognize brilliant ideas, interpret brilliant ideas, develop brilliant ideas.
You might want to follow his example in your own work life: don’t be too quick to take the credit; instead, let your co-workers come to the realization that good things always seem to happen when you’re around.
Of course, there were two components to extraordinary things always seeming to happen when John von Neumann was around.
One was his being brilliant.
The other was his being there.
Being in the right place at the right time isn’t luck. At least, it’s not luck if you do it as consistently as John von Neumann did it.
Being in the right place at the right time is an art.
People always wanted John von Neumann around. They invited him to prestigious institutions. They involved him in innovative projects. They wanted to discuss their most profound ideas with him. And, across an extraordinarily diverse range of fields, he was always interested.
John von Neumann’s life is a masterclass in the art of being there.
—
Images
- Image of John von Neumann from the Los Alamos National Laboratory, which rather pointlessly requires that this rather ponderous statement be reproduced here: “Unless otherwise indicated, this information has been authored by an employee or employees of the Los Alamos National Security, LLC (LANS), operator of the Los Alamos National Laboratory under Contract No. DE-AC52-06NA25396 with the U.S. Department of Energy. The U.S. Government has rights to use, reproduce, and distribute this information. The public may copy and use this information without charge, provided that this Notice and any statement of authorship are reproduced on all copies. Neither the Government nor LANS makes any warranty, express or implied, or assumes any liability or responsibility for the use of this information.”
- Turing Machine Model Davey 2012 by Rocky Acosta licensed under CC BY 3.0
- Bundesarchiv Bild183-R57262, Werner Heisenberg by an unknown author (Bundesarchiv, Bild 183-R57262) licensed under CC BY-SA 3.0 DE
- Turing in 1935 by Tomipelegrin licensed under CC BY-SA 4.0
- Gospers glider gun by Lucas Vieira licensed under CC BY-SA 3.0